Advertisements
Advertisements
Question
In ΔABC right angled at B, ∠A = ∠C. Find the value of:
(i) sinA cosC + cosA sinC
(ii) sinA sinB + cosA cosB
Solution
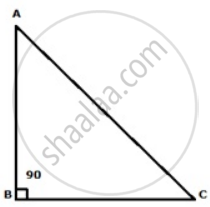
Since ∠B is right angled ⇒ ∠B = 90°
In ΔABC,
∠A + ∠B + ∠C = 180°
But ∠A = ∠C
⇒ ∠A + 90° + ∠A = 180°
⇒ 2∠A = 90°
⇒ ∠A = 45° = ∠C
(i) sinA cosC + cosA sinC
= sin45° cos45° + cos45° sin45°
= `(1)/sqrt(2) xx (1)/sqrt(2) + (1)/sqrt(2) xx (1)/sqrt(2)`
= `(1)/(2) + (1)/(2)`
= 1
(ii) sinA sinB + cosA cosB
sin45° sin90° + cos45° cos90°
= `(1)/sqrt(2) xx 1 + (1)/sqrt(2) xx 0`
= `(1)/sqrt(2)`.
APPEARS IN
RELATED QUESTIONS
Evaluate the following in the simplest form:
sin 60° cos 30° + cos 60° sin 30°
If θ is an acute angle and sin θ = cos θ, find the value of 2 tan2 θ + sin2 θ – 1
Evaluate cos 48° − sin 42°
Evaluate the following
`sec 11^@/(cosec 79^@)`
Evaluate the following :
`((sin 49^@)/(cos 41^@))^2 + (cos 41^@/(sin 49^@))^2`
Express each one of the following in terms of trigonometric ratios of angles lying between 0° and 45°
cot 85° + cos 75°
If A, B, C are the interior angles of a triangle ABC, prove that
`tan ((C+A)/2) = cot B/2`
Prove that sin 48° sec 42° + cos 48° cosec 42° = 2
Prove that
cosec (67° + θ) − sec (23° − θ) = 0
Express each of the following in terms of trigonometric ratios of angles lying between 0° and 45°.
cosec54° + sin72°
find the value of: sin 30° cos 30°
Evaluate:
`(cos3"A" – 2cos4"A")/(sin3"A" + 2sin4"A")` , when A = 15°
Given A = 60° and B = 30°,
prove that : sin (A + B) = sin A cos B + cos A sin B
Prove that:
cosec2 45° - cot2 45° = 1
Prove that:
cos2 30° - sin2 30° = cos 60°
ABC is an isosceles right-angled triangle. Assuming of AB = BC = x, find the value of each of the following trigonometric ratios: tan 45°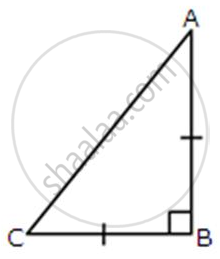
If A =30o, then prove that :
cos 2A = cos2A - sin2A = `(1 – tan^2"A")/(1+ tan^2"A")`
Without using tables, evaluate the following: sec30° cosec60° + cos60° sin30°.
Without using tables, evaluate the following: tan230° + tan260° + tan245°
Find the value of x in the following: 2 sin3x = `sqrt(3)`
Find the value of x in the following: `sqrt(3)sin x` = cos x
If A = 30° and B = 60°, verify that: `(sin("A" -"B"))/(sin"A" . sin"B")` = cotB - cotA
If tan(A - B) = `(1)/sqrt(3)` and tan(A + B) = `sqrt(3)`, find A and B.
Prove the following:
`(sqrt(3) + 1) (3 - cot 30^circ)` = tan3 60° – 2 sin 60°
The value of 5 sin2 90° – 2 cos2 0° is ______.
`(2/3 sin 0^circ - 4/5 cos 0^circ)` is equal to ______.
Evaluate: sin2 60° + 2tan 45° – cos2 30°.
