Advertisements
Advertisements
Question
Without using tables, evaluate the following: 4(sin430° + cos460°) - 3(cos245° - sin290°).
Solution
4(sin430° + cos460°) - 3(cos245° - sin290°).
sin30° = `(1)/(2)`
sin90° = 1
cos45° = `(1)/sqrt(2)`
cos60° = `(1)/(2)`
4(sin430° + cos460°) - 3(cos245° - sin290°)
= `4((1/2)^4 + (1/2)^4) -3((1/sqrt(2))^2 - (1)^2)`
= `4(1/16 + 1/16) -3(1/2 - 1)`
= `4 xx (2)/(16) + 3 xx (1)/(2)`
= `(1)/(2) + (3)/(2)`
= `(4)/(2)`
= 2.
APPEARS IN
RELATED QUESTIONS
If x = 30°, verify that
(i) `\tan 2x=\frac{2\tan x}{1-\tan ^{2}x`
(ii) `\sin x=\sqrt{\frac{1-\cos 2x}{2}}`
If θ is an acute angle and sin θ = cos θ, find the value of 2 tan2 θ + sin2 θ – 1
If tan (A + B) = `sqrt3` and tan (A – B) = `1/sqrt3`; 0° < A + B ≤ 90°; A > B, find A and B.
Evaluate the following:
`(sin 30° + tan 45° – cosec 60°)/(sec 30° + cos 60° + cot 45°)`
State whether the following is true or false. Justify your answer.
sinθ = cosθ for all values of θ.
Evaluate the following :
`(sin 21^@)/(cos 69^@)`
Evaluate the following :
`tan 10^@/cot 80^@`
Evaluate the following :
(sin 72° + cos 18°) (sin 72° − cos 18°)
Evaluate the following :
sin 35° sin 55° − cos 35° cos 55°
Evaluate the following
sec 50º sin 40° + cos 40º cosec 50º
Express each one of the following in terms of trigonometric ratios of angles lying between 0° and 45°
tan 65° + cot 49°
Express each one of the following in terms of trigonometric ratios of angles lying between 0° and 45°
sin 67° + cos 75°
Prove the following
sin θ sin (90° − θ) − cos θ cos (90° − θ) = 0
Evaluate:
`(cos3"A" – 2cos4"A")/(sin3"A" + 2sin4"A")` , when A = 15°
find the value of :
3sin2 30° + 2tan2 60° - 5cos2 45°
Prove that:
cosec2 45° - cot2 45° = 1
Prove that:
`((tan60° + 1)/(tan 60° – 1))^2 = (1+ cos 30°) /(1– cos 30°) `
ABC is an isosceles right-angled triangle. Assuming of AB = BC = x, find the value of each of the following trigonometric ratios: tan 45°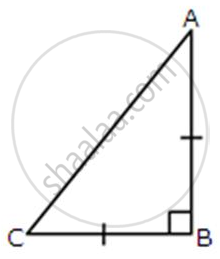
Without using tables, evaluate the following: sec30° cosec60° + cos60° sin30°.
Find the value of x in the following: cos2x = cos60° cos30° + sin60° sin30°
If A = B = 45°, verify that cos (A − B) = cos A. cos B + sin A. sin B
In ΔABC right angled at B, ∠A = ∠C. Find the value of:
(i) sinA cosC + cosA sinC
(ii) sinA sinB + cosA cosB
Verify the following equalities:
1 + tan2 30° = sec2 30°
If 2 sin 2θ = `sqrt(3)` then the value of θ is
If sin(A + B) = 1 and cos(A – B)= `sqrt(3)/2`, 0° < A + B ≤ 90° and A > B, then find the measures of angles A and B.
Find the value of x if `2 "cosec"^2 30 + x sin^2 60 - 3/4 tan^2 30` = 10
Evaluate: sin2 60° + 2tan 45° – cos2 30°.
