Advertisements
Advertisements
प्रश्न
Advertisements
उत्तर
We have,
\[\frac{dy}{dx} = \tan^{- 1} x\]
\[ \Rightarrow dy = \left( \tan^{- 1} x \right)dx\]
Integrating both sides, we get
\[\int dy = \int\left( \tan^{- 1} x \right)dx\]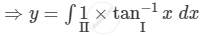
\[ \Rightarrow y = \tan^{- 1} x\int1 dx - \int\left[ \frac{d}{dx}\left( \tan^{- 1} x \right)\int1 dx \right]dx\]
\[ \Rightarrow y = x \tan^{- 1} x - \int\frac{x}{1 + x^2}dx\]
\[ \Rightarrow y = x \tan^{- 1} x - \frac{1}{2}\int\frac{2x}{1 + x^2}dx\]
\[ \Rightarrow y = x \tan^{- 1} x - \frac{1}{2}\log\left| 1 + x^2 \right| + C\]
\[\text{ So, } y = x \tan^{- 1} x - \frac{1}{2}\log\left| 1 + x^2 \right| +\text{C is defined for all }x \in R.\]
\[\text{ Hence, } y = x \tan^{- 1} x - \frac{1}{2}\log\left| 1 + x^2 \right| +\text{C is the solution to the given differential equation.}\]
APPEARS IN
संबंधित प्रश्न
If 1, `omega` and `omega^2` are the cube roots of unity, prove `(a + b omega + c omega^2)/(c + s omega + b omega^2) = omega^2`
Find the differential equation of all the parabolas with latus rectum '4a' and whose axes are parallel to x-axis.
Show that the differential equation of which y = 2(x2 − 1) + \[c e^{- x^2}\] is a solution, is \[\frac{dy}{dx} + 2xy = 4 x^3\]
For the following differential equation verify that the accompanying function is a solution:
| Differential equation | Function |
|
\[x\frac{dy}{dx} + y = y^2\]
|
\[y = \frac{a}{x + a}\]
|
Differential equation \[\frac{dy}{dx} + y = 2, y \left( 0 \right) = 3\] Function y = e−x + 2
(y2 + 1) dx − (x2 + 1) dy = 0
Solve the differential equation \[\frac{dy}{dx} = \frac{2x\left( \log x + 1 \right)}{\sin y + y \cos y}\], given that y = 0, when x = 1.
The surface area of a balloon being inflated, changes at a rate proportional to time t. If initially its radius is 1 unit and after 3 seconds it is 2 units, find the radius after time t.
The rate of growth of a population is proportional to the number present. If the population of a city doubled in the past 25 years, and the present population is 100000, when will the city have a population of 500000?
In a culture, the bacteria count is 100000. The number is increased by 10% in 2 hours. In how many hours will the count reach 200000, if the rate of growth of bacteria is proportional to the number present?
The rate of increase in the number of bacteria in a certain bacteria culture is proportional to the number present. Given the number triples in 5 hrs, find how many bacteria will be present after 10 hours. Also find the time necessary for the number of bacteria to be 10 times the number of initial present.
If the marginal cost of manufacturing a certain item is given by C' (x) = \[\frac{dC}{dx}\] = 2 + 0.15 x. Find the total cost function C (x), given that C (0) = 100.
A curve is such that the length of the perpendicular from the origin on the tangent at any point P of the curve is equal to the abscissa of P. Prove that the differential equation of the curve is \[y^2 - 2xy\frac{dy}{dx} - x^2 = 0\], and hence find the curve.
The slope of a curve at each of its points is equal to the square of the abscissa of the point. Find the particular curve through the point (−1, 1).
The integrating factor of the differential equation \[\left( 1 - y^2 \right)\frac{dx}{dy} + yx = ay\left( - 1 < y < 1 \right)\] is ______.
Solve the following differential equation : \[y^2 dx + \left( x^2 - xy + y^2 \right)dy = 0\] .
Verify that the function y = e−3x is a solution of the differential equation \[\frac{d^2 y}{d x^2} + \frac{dy}{dx} - 6y = 0.\]
In the following verify that the given functions (explicit or implicit) is a solution of the corresponding differential equation:-
y = ex + 1 y'' − y' = 0
Form the differential equation of the family of parabolas having vertex at origin and axis along positive y-axis.
Choose the correct option from the given alternatives:
The solution of `1/"x" * "dy"/"dx" = tan^-1 "x"` is
Find the differential equation whose general solution is
x3 + y3 = 35ax.
The solution of `dy/dx + x^2/y^2 = 0` is ______
Choose the correct alternative.
Bacteria increases at the rate proportional to the number present. If the original number M doubles in 3 hours, then the number of bacteria will be 4M in
A solution of a differential equation which can be obtained from the general solution by giving particular values to the arbitrary constants is called ___________ solution.
x2y dx – (x3 + y3) dy = 0
`dy/dx = log x`
Select and write the correct alternative from the given option for the question
Bacterial increases at the rate proportional to the number present. If original number M doubles in 3 hours, then number of bacteria will be 4M in
The solution of differential equation `x^2 ("d"^2y)/("d"x^2)` = 1 is ______
State whether the following statement is True or False:
The integrating factor of the differential equation `("d"y)/("d"x) - y` = x is e–x
Verify y = log x + c is the solution of differential equation `x ("d"^2y)/("d"x^2) + ("d"y)/("d"x)` = 0
Integrating factor of the differential equation `x "dy"/"dx" - y` = sinx is ______.
Solution of `x("d"y)/("d"x) = y + x tan y/x` is `sin(y/x)` = cx
There are n students in a school. If r % among the students are 12 years or younger, which of the following expressions represents the number of students who are older than 12?
